|
Why stars don't shine at daytime ?
As
often on Internet forums, at the question "why stars are
not visible at daytime" posted by an amateur it is not enough to answer "because the sun
shines, guy... !".
The eye and CCD
It
is in reading technical notes about CCD's, that I had the idea
to compare the signal-to-noise of this electronic device with
the reponse of the human eye, what helped me to answer to the
above question.
In summary the problem of seeing a star in daylight in linked to the sensitivity
of the eye fovea and to the level of influence of the parasitic light
presents in the observer near-environment.
What, at first sight, can be considered as a
"filter" caused by the light spread in the atmosphere can be
translated by the "noise" generated by the sky background, in this case the presence of
the intense sunlight. How to explain this phenomenon in
technical and mathematical terms and link it to the signal-to-noise ratio ? Here is
a explanation.
In the past I was pro photographer and user of image processing technics to
enhance my B/W or colors snapshots (including astronomy pics) as my teachers
always asked me to do (at least in the beginning) : "balance this color,
reduce this dominence, reveales more details in the light please",... I
learnt the lesson ! I used masks, sandwitches of negatives, etc to reach this
objective. Now thing changed. I am pro computist, my interest in
astrophotography remains but the problem became more technical. We use
computers, image intensifiers, CCD detectors and we try to reduce their
"quantum defaults" in order to get good pictures, well balanced for
colors, saturated, with the lesser noise of all kind we can... In short, a true
challenge !
Made a comparison between the human eye and a
CCD device. As you know both are light detectors that have the ability to
process a continue analog signal. The CCD transforms photons in digital
data (electrons are converted in binary digits) that we can easily process using
a computer as they "speak" the same binary language.
|
Astronomers'
eyes |
|

|

|
|
To
understand why stars are not visible at daytime, it is
useful to compare the human eye with an electronic
detector like a CCD. See the text for explanations. |
|
The sensitivity of our eye, as the one of a CCD detector depends, besides
its specifications, on how we (our
brain or the image acquisition software) process the "noises" present
in the raw original signal.
As we explained in the chapter dealing with CCD cameras,
these "noises" could be the readout noise (parasits introduced during
the A/D conversion), the dark current and bias caused by the electron agitation
(temperature) in a CCD and the background noise coming from natural and
artificial sources (skyglow, moonlight, light pollution...). The eye is not a
electronic device but it takes advantage of the electromagnetic properties of
electrons when it has to transmit its information to the brain via the optic
nerve. Now what is the relation between the eye and the CCD in this matter ?
I'am not a neuro-physicist (the eyes are "only" brain extensions) nor
a cybernetician, but the "readout noise" and the "dark
current" are probably reduced to nothing in our eyes (the brain). Without
stimulus, we detect no visual signal and we stay like blinded... But
whatever their form, if these signals exist you will quickly understand by
reading the remain explanation their contribution is negligible.
Rest the "background noise". At night it is surely not negligible even
if we substact the liners and other jets that cross through the firmament. This
"noise" can for example appear when a
cosmic ray hits one of our sensitive eye cells or a neural one. In these rare
occasions we could see a flash light in our eyes like an instantaneous nova
lighting the night. But it really appears in skyglow (excited atoms emitting
light) and light pollution which
limit our ability to see faint objects (in urban sites the signal and noise can
be on par, offering us few chance to see stars).
To be complete we have to add
the weather factor too. But if the seeing and transparency are at their best, the source signal (planet, moon, star, DSO) is
stronger than the noise. This explanation with ordinary words help us to
understand why we can see stars in the night; "noises" of all kind have intensities much
lower than signal coming from stars.
But what's the matter at daytime ?
Maths
and physics
Trying to find a star in daylight,
we have to admit that conditions are completely different and opposite to the
night conditions. At first sight we can say now the Sun and the sky brightness add
much "noises" over the star signal, and not simply a "filter" we
could substract from raws images. Yes, this is the explanation ! Now the mathematical
explanation.
Imagine a thinking experiment (all numbers are fictive, just for the demonstration)
in which we want to look at
a star in the daylight. The sunlight, the potential star light and noises of all
kind hit our sensitives eye cells at a rate of 10000 counts per second per cell.
This value represents the source signal without discriminating the star one.
Remember too the field of view of the eye is around 120 degrees wide, so
potentially catching a wide area of the sky and much light, probably adding a brightness factor
we'll confirm later
Such a signal is a complex entity, composed of wavefronts
of photon, quanta of energy "rule" by the quantum theory. The noise is
thus a random quantum event according this theory. As for a CCD detector, the
total noise (N) presents in the signal we search for naked eye represents the
quantum uncertainty or the standard deviation from the average brightness. Its
expression is defined as the square root of the sum of the squares of the
individual noises values (oops !) :
N = Ö¯(
noise12 + noise22 +...)
<Equation 1>
What becomes the star signal now ? Comparing with a night measurement focused on
this peculiar star, we know after integration that in the incoming daylight
signal, our sensitive eye cells count to say, 50 hits due to the star. We know
the star signal is defined as the input signal without the sun and other noises
contributions. All being an affair of random quantum events, the contribution of
all noises (equation 1) produces :
Ö¯10000, or 100 hits per
visual cell.
Now knowing that the 50 hits from the star are mixed with the 9950 others due to
the combination of sunlight, skylow and other light pollution spread in the field, we can determine the power of the star signal, the signal-to-noise
ratio as one say, or S/N. Applying our relations to the star, S/N = 50/100, or 0.5. What means
this number ?
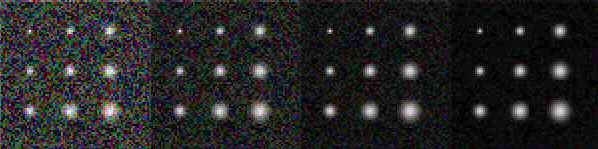
|
|
|
Upper,
simulation on stars of increasing magnitudes of a
signal-to-noise ratio ranging from 2:1 to 16:1. Below the same
simulation tested on an artificial nebula. Documents Kazuyuki
Tanaka. |
|

|
|
In this example
0.5 means that the star magnitude (or brightness) can not be seen
with a precision better than 1/0.5, or a 2 factor !, a value at least 10 times
less than the accepted thresold of detectability for a CCD detector (which S/N
may reaches 20 or more per pixel, able to detect a 20st magnitude star per
arcsecond squared), but our eye is not exactly a CCD as our sensitives eye cells
cannot accumulate light ! This value of 0.5 means also the star brightness is
embedded is the sky brightness which considerably decreases the signal-to-noise
ratio of the star we try to detect in the daylight. Exposed on a monitor, the
image of our star is nearly invisible in the sky brightness given the feeling of looking
at an old TV screen full of parasites.
Now we can solve our problem of trying to see a star in presence of the Sun. How
to proceed ? Simply in increasing the S/N ratio of the star. How ? If we reduce
our field of view using for example a scope or a long tube providing a true
field of view of around 10' or less we reduce drastically the noise contribution
of a ten factor or more and increase our star S/N ratio. Using this
construction, the S/N ratio of the star is now enhanced while the background
brightness is strongly reduced. On our control monitor the image is became more soft,
the star appears now like a small bright dot circled by a dim blue-grey skyglow,
typical of a lower signal deviation from the average or, in other words, a smaller
S/N uncertainty.
By this thinking construction we have reduced noises contributions in our
star S/N ratio to 50/Ö¯1000 or less in place of
50/Ö¯10000, so around 15 or better in place of 0.5. With this
"sampling" value 30 times higher we can theoretically discriminate
more easly the star signal, thus see some stars at daytime and estimate their
magnitude with a precision of 1/15 or around 7% in place of a 2 factor !
Conclusion
With words borrowed to the CCD world, we can say that at start our star image was
"undersampled" and cannot be recorded by our visual detector (eye). To
enhance the "sampling" and discrimate our star in the daylight, we
have had to increase the "scope" focal length to get a larger image
scale. But the comparison stops here as we cannot use terms as focal reducer or
binning (combination of pixels) to reach this specific goal, as we do using a
CCD. But I never say we cannot substrat the sky brightness and increase this way
the star signal using a CCD and image processing software. Don't interpret my
words…
Thanks maths !
Back
to Reports & Reviews
|